Acoustics of Sound-holes in Musical Instruments
The present day violin is acoustically so sophisticated that 200 years of scientific study has not been able to systematically distinguish between a good and an average violin. The present-day violin making is based on replicating old Italian violins widely accepted as perfect instruments; a perfection that is reached only by hands of artist, and not yet reproducible by quantitative scientific methods. One of the major questions is the driving forces in the evolution of f-hole’s geometrical design that has ended up to the present-day f-shape. The main contribution of f-hole in violin is to amplify the sound radiation (air resonance) at low frequencies, at which the soundboard is inefficient in sound radiation.
The efficiency of sound radiation at low frequencies in musical instruments is improved by the use of a sound hole. The sound hole enhances the radiation of sound by vibrating the volume of air inside as well as near its opening. A common example is an empty bottle: the air inside vibrates when you blow across the top, and enhances the radiated sound. This phenomenon, called the Helmholtz resonance, is utilized in musical instruments to enhance the radiation of sound at low frequencies.
An important characteristic of the sound-hole is the frequency at which the radiated sound is enhanced the most. This frequency, called the resonance frequency, can easily be obtained for holes with simple geometries such as the circular hole in guitar (see The Theory of Sound, vol. 2 by Lord Rayleigh). For the sound holes with complicated shapes such as f-holes or lute rosettes (See below), finding the resonance characteristics is much more challenging.
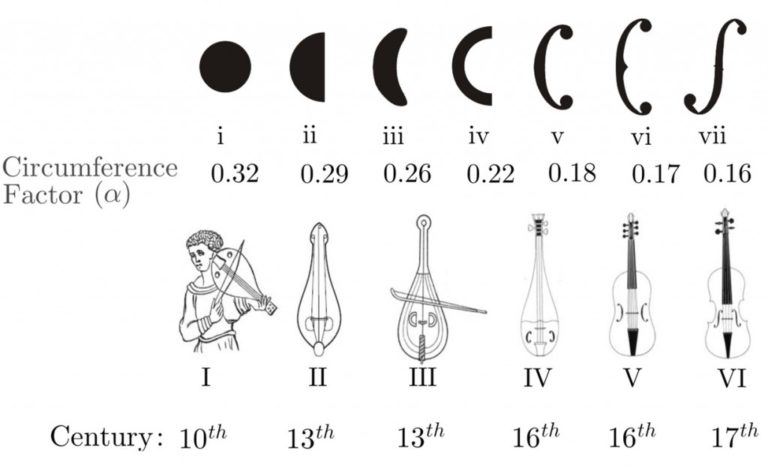
Utilizing experimental and numerical simulation techniques, we showed that the evolution of violin moves toward acoustic power efficiency: for a constant void area, f-hole has the maximum power radiation among other historic soundhole geometries in the violin family: circular opening (10th-century viols), semi-circular opening (Lyras in 13th century), crescent and semi-circular strip (13th century), primary c-hole and c-hole (early viols and violins in 16th century), and present-day f-hole in violins (unchanged since 17th century).
The historic developmental path from circular soundhole to the present-day f-hole goes toward maximizing radiation at air resonance for a fixed void area. Comparing an f-hole to a circular soundhole with the same void area, the resonance frequency, f, of the f-hole differs from the one of the circular by 4 semitones. Treating the radiation of sound during resonance as a monopole, the radiating power W has a power law relationship with the resonance frequency as , which results in 4.5 dB higher radiating power in f-hole compared to circular opening with the same area. Our method of analysis was verified by measuring the resonance and bandwidth of different openings on a standard resonance box (see Fig below)
Thanks to Johnson String Instrument we had the chance to verify our results experimentally on a number of very good instruments:
References:
– Tavakoli Nia, H., Alam, M.-R., Liu, Y., and Makris, N., “Acoustics efficiency of Soundholes in Musical Instrument”. To be submitted.